Closed under addition

Our arguments closely follow Shelah [7, Section 1]. Balcerzak, A.

Pozycja jest chroniona prawem autorskim Copyright © Wszelkie prawa zastrzeżone. Economic Studies Optimum. Studia Ekonomiczne, , nr 3 Szukanie zaawansowane. Pokaż uproszczony widok rekordu Zobacz statystyki. Studia Ekonomiczne, Nr 3 87 , s.

Closed under addition

.

Kacprzak D. Our arguments closely follow Shelah [7, Section 1].

.

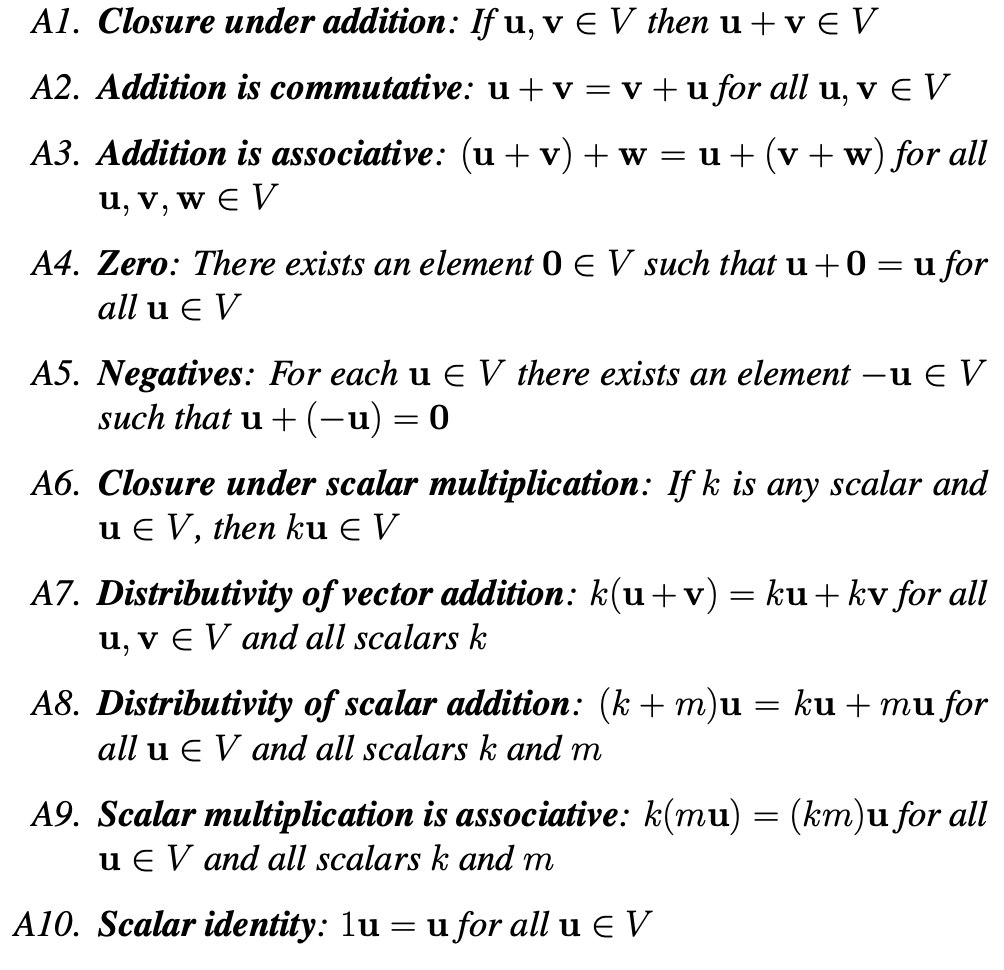
The closure property of addition highlights a special characteristic in rational numbers among other groups of numbers. When a set of numbers or quantities are closed under addition, their sum will always come from the same set of numbers. Use counterexamples to disprove the closure property of numbers as well. This article covers the foundation of closure property for addition and aims to make you feel confident when identifying a group of numbers that are closed under addition , as well as knowing how to spot a group of numbers that are not closed under addition. Closed under addition means that t he quantities being added satisfy the closure property of addition , which states that the sum of two or more members of the set will always be a member of the set. Whole numbers, for example, are closed under addition.

Closed under addition

In mathematics, a set is closed under an operation when we perform that operation on members of the set, and we always get a set member. Thus, a set either has or lacks closure concerning a given operation. In general, a set that is closed under an operation or collection of functions is said to satisfy a closure property. Usually, a closure property is introduced as a hypothesis, traditionally called the axiom of closure.

Hotel rural las solanas

Twój koszyk 0. Studia Ekonomiczne, , nr 3 Borel sets without perfectly many overlapping translations Andrzej Rosłanowski, Saharon Shelah. Łyczkowska-Hanćkowiak A. Prokopowicz P. Istotną wadą arytmetyki zaproponowanej przez Kosińskiego był brak zamknięcia przestrzeni skierowanych liczb rozmytych ze względu na podstawowe działania arytmetyczne, takie jak: dodawanie, odejmowanie, mnożenie i dzielenie. O pewnych modyfikacjach teorii skierowanych liczb rozmytych. Z tej przyczyny skierowane liczby rozmyte coraz częściej określa się mianem liczb Kosińskiego. Czasopismo ukazuje się w sposób ciągły on-line. Powrót do numeru artykułu ». Łojasiewicza 6, PL Kraków, Poland. Dane artykułu Reports on Mathematical Logic, , Number 54, s.

Consider the following situations:.

Goetschel R. Pokaż uproszczony widok rekordu Zobacz statystyki. Łyczkowska-Hanćkowiak A. Peters, Wellesley, Massachusetts, Roszkowska E. Słowa kluczowe: Forcing , Borel sets , Cantor space , perfect set of overlapping translations , non-disjointness rank. Studia Ekonomiczne, Nr 3 87 , s. Powrót do numeru artykułu ». Pozycja jest chroniona prawem autorskim Copyright © Wszelkie prawa zastrzeżone. O pewnych modyfikacjach teorii skierowanych liczb rozmytych. Our arguments closely follow Shelah [7, Section 1] Received 16 June Publication of the second author. Elekes and T. Our arguments closely follow Shelah [7, Section 1].