Common chord of two circles formula

Thus, this is precisely the common chord! The length of the common chord can be easily evaluated using the Pythagoras theorem:. Now an interesting question arises.

Hopefully I am thinking of the easiest way to solve the problem, but start by drawing the following diagram:. You can see the scalene triangle ABC in this diagram. Let's now redraw this without the circles:. You should recognize that the chord and the radial line AB are perpendicular, so a is the height of triangle ABC and d is its base. You can re-arrange each of the circle equations into standard circle form which allows you to read off the radius and the center position of each circle. Assign one radius as R and the other as r. Use the distance formula to calculate the distance between the two centers that's d.

Common chord of two circles formula

.

Online Tutors.

.

The chord of a circle can be stated as a line segment joining two points on the circumference of the circle. The diameter is the longest chord of the circle which passes through the center of the circle. The figure shown below represents the circle and its chord. In the circle above with center O, AB represents the diameter of the circle longest chord of a circle , OE represents the radius of a circle and CD represents the chord of a circle. Let us consider CD as the chord of a circle and points P and Q lying anywhere on the circumference of the circle.

Common chord of two circles formula

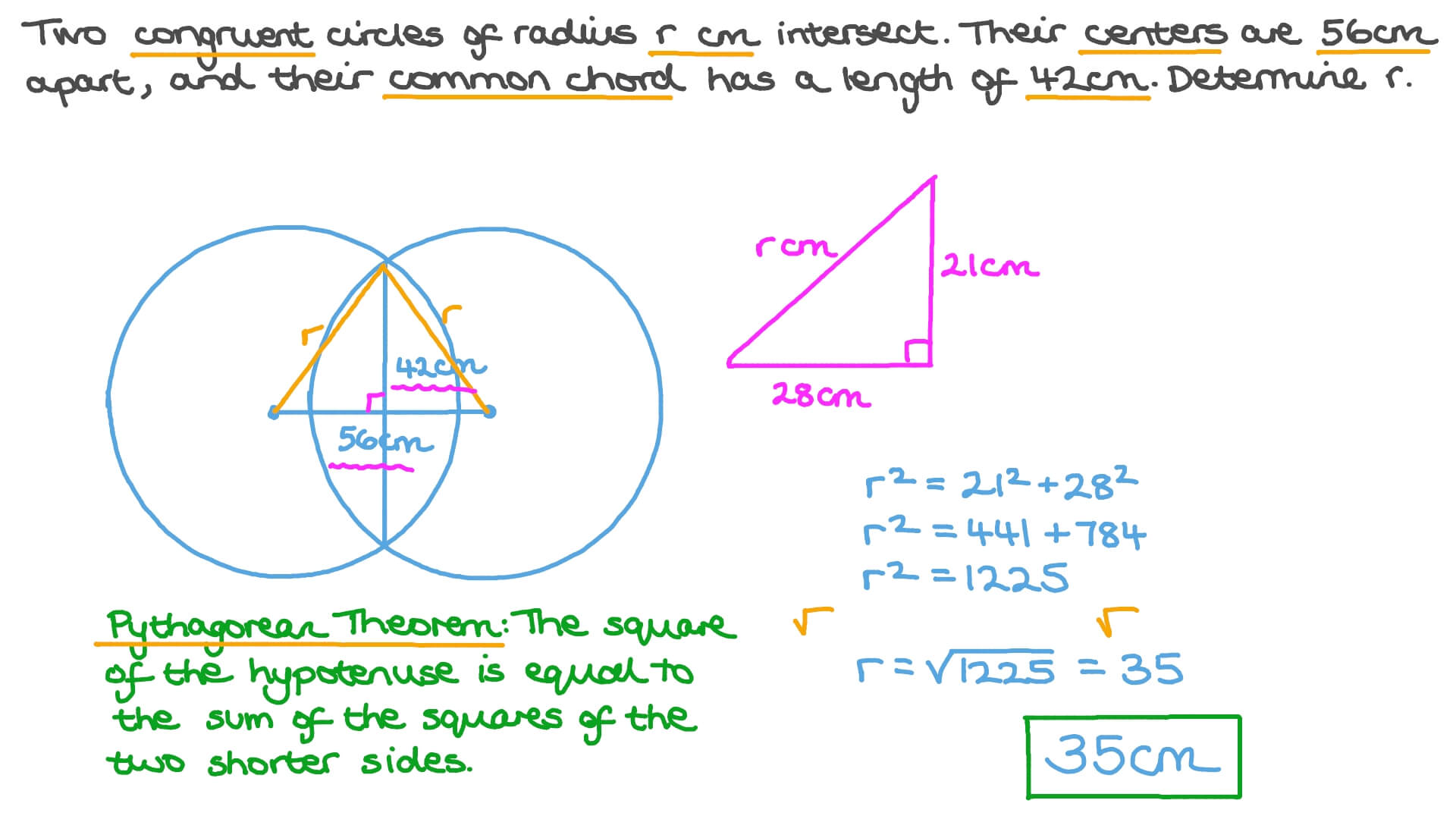
If we know the radii of two intersecting circles, and how far apart their centers are, we can calculate the length of the common chord. Circles O and Q intersect at points A and B. The radius of circle O is 16, and the radius of circle Q is 9. Line OQ connects the centers of the two circles and is 20 units long. Find the length of the common chord AB. We know that line OQ is the perpendicular bisector of the common chord AB. And we are also given the lengths of the radii, so we probably need to use that. Let's draw these radii:. They are both right triangles since OQ is perpendicular to AB , and both have the same height, h.

Earthquake today camarines sur

Terms and Conditions. Kindergarten Worksheets. Already booked a tutor? The height of a triangle times half its base is the area of the triangle. It should be obvious that in case of intersecting or touching circles, the common chord or the common tangent is itself the radical axis. Book a Free Class. What does this equation tell us? Maths Games. Let's now redraw this without the circles:. Hopefully I am thinking of the easiest way to solve the problem, but start by drawing the following diagram:. Multiplication Tables. Online Tutors.

Now we need to find the equation of the common chord PQ of the given circles. Now subtracting the equation 4 from equation 3 we get,.

Practice worksheets in and after class for conceptual clarity. The angle of intersection of the two circles can be defined as the angle between the tangents to the two circles at their point s of intersection, which will be the same as the angle between the two radii at the point s of intersection. Common Chords And Radical Axes. Learn from the best math teachers and top your exams. Maths Puzzles. Let's now redraw this without the circles: You should recognize that the chord and the radial line AB are perpendicular, so a is the height of triangle ABC and d is its base. For a situation as in Fig - 37 above, the radical axis exists but no common chord exists. Hi Shubha. Kindergarten Worksheets. United States. Let's now redraw this without the circles:.