Integral of secx

To find the integral of sec x, we will have to use some facts from trigonometry. We can do the integration of secant x in multiple methods such as:.

In calculus , the integral of the secant function can be evaluated using a variety of methods and there are multiple ways of expressing the antiderivative , all of which can be shown to be equivalent via trigonometric identities ,. This formula is useful for evaluating various trigonometric integrals. In particular, it can be used to evaluate the integral of the secant cubed , which, though seemingly special, comes up rather frequently in applications. The integral of the secant function was historically one of the first integrals of its type ever evaluated, before most of the development of integral calculus. It is important because it is the vertical coordinate of the Mercator projection , used for marine navigation with constant compass bearing. The integral of the secant function was one of the "outstanding open problems of the mid-seventeenth century", solved in by James Gregory.

Integral of secx

.

Translated by Child, James Mark. What is the Integration of Sec x? Integral of Sec x by Hyperbolic Functions 6.

.

In this section we look at how to integrate a variety of products of trigonometric functions. These integrals are called trigonometric integrals. They are an important part of the integration technique called trigonometric substitution , which is featured in Trigonometric Substitution. This technique allows us to convert algebraic expressions that we may not be able to integrate into expressions involving trigonometric functions, which we may be able to integrate using the techniques described in this section. In addition, these types of integrals appear frequently when we study polar, cylindrical, and spherical coordinate systems later. For integrals of this type, the identities. After applying these formulas, simplify and reapply strategies 1 through 3 as appropriate. Use strategy 2. These formulas may be derived from the sum-of-angle formulas for sine and cosine.

Integral of secx

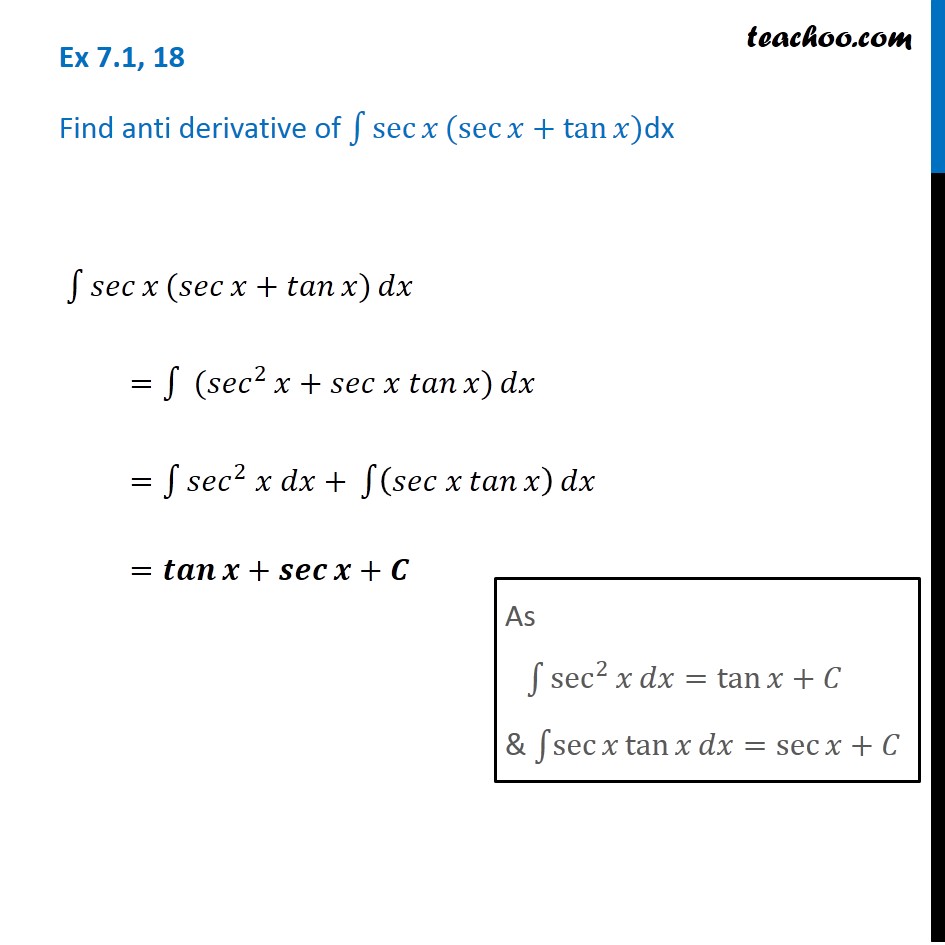
To find the integral of sec x, we will have to use some facts from trigonometry. We can do the integration of secant x in multiple methods such as:. We have multiple formulas for integration of sec x and let us derive each of them using the above mentioned methods. Also, we will solve some examples related to the integral of sec x. This is also known as the antiderivative of sec x. We have multiple formulas for this. Here "ln" stands for natural logarithm and 'C' is the integration constant. Multiple formulas for the integral of sec x are listed below:. We can find the integral of sec x by substitution method. But why do we need to do this?

Castillo casper

This conjecture became widely known, and in , Isaac Newton was aware of it. Moses Pitt. United Kingdom. Thus, the integral of sec x tan x is sec x. We have multiple formulas for this. The Geometrical Lectures of Isaac Barrow. Our Journey. Differentiation rules List of integrals of exponential functions List of integrals of hyperbolic functions List of integrals of inverse hyperbolic functions List of integrals of inverse trigonometric functions List of integrals of irrational functions List of integrals of logarithmic functions List of integrals of rational functions List of integrals of trigonometric functions Secant Secant cubed List of limits Lists of integrals. Saudi Arabia. Fractional Malliavin Stochastic Variations.

There are of course a very large number 1 of trigonometric identities, but usually we use only a handful of them.

Maths Program. Sri Lanka. This substitution can be obtained from the derivatives of secant and tangent added together, which have secant as a common factor. We know that the derivative of sec x is sec x tan x. Advanced Calculus on Euclidean space Generalized functions Limit of distributions. Conformal Projections Based on Elliptic Functions. Maths Games. Lists of integrals Integral transform Leibniz integral rule Definitions Antiderivative Integral improper Riemann integral Lebesgue integration Contour integration Integral of inverse functions Integration by Parts Discs Cylindrical shells Substitution trigonometric , tangent half-angle , Euler Euler's formula Partial fractions Changing order Reduction formulae Differentiating under the integral sign Risch algorithm. Limits Continuity. Fractional Malliavin Stochastic Variations. Indulging in rote learning, you are likely to forget concepts. A similar strategy can be used to integrate the cosecant , hyperbolic secant , and hyperbolic cosecant functions. The integral of the secant function was historically one of the first integrals of its type ever evaluated, before most of the development of integral calculus. Maths Questions. Now, we have.