Lcm in division method

Both the methods are explained here with many examples. We have provided the prime factors of the given numbers, such as 24, 12, 30,etc.

For two integers a and b, denoted LCM a,b , the LCM is the smallest positive integer that is evenly divisible by both a and b. The LCM of two or more numbers is the smallest number that is evenly divisible by all numbers in the set. Find the LCM of a set of numbers with this calculator which also shows the steps and how to do the work. Input the numbers you want to find the LCM for. You can use commas or spaces to separate your numbers. But do not use commas within your numbers.

Lcm in division method

LCM by division method means finding the least common multiple of a given set of numbers by dividing all the given numbers by a common prime number. This is one of the easiest methods to find the LCM of numbers. Let us learn about the LCM by division method on this page. This method of finding the LCM of numbers is one of the most common methods which gives the result quickly. For this, we need to know the common multiplication tables and those prime numbers that can divide the given numbers completely. In order to find the LCM by the common division method, we need to know the prime factors of the given numbers. Let us see the how to calculate the LCM of 24, 36, and 48 using the division method using the steps given below:. In order to find the LCM by division method, we use the same steps given above. However, if we need to find the HCF by long division method, it has a different working. Let us understand this using an example for both. Solution: First let us find the LCM of 12 and 18 using the steps discussed below. It should be noted that the HCF of 12 and 18 is the divisor that we get when the remainder becomes 0 after doing long division repeatedly. Solution: The LCM by division method can be calculated using the following steps. In order to find the LCM by division method, we find a common prime number that divides the given numbers.

People use the cake or ladder method as the fastest and easiest way to find the LCM because it is simple division.

LCM by division is the process of finding the least common multiple of a given group of integers by dividing them by a common prime number. For this, we need to completely understand the prime factors of the given numbers. Prime factorization and listing the multiples are two additional techniques for calculating LCM. This technique of determining the LCM of numbers is one of the standards and provides a rapid result. We must understand the common multiplication tables and the prime numbers that can totally divide the provided values. To obtain the LCM using the common division method, we must first determine the prime factors of the provided integers.

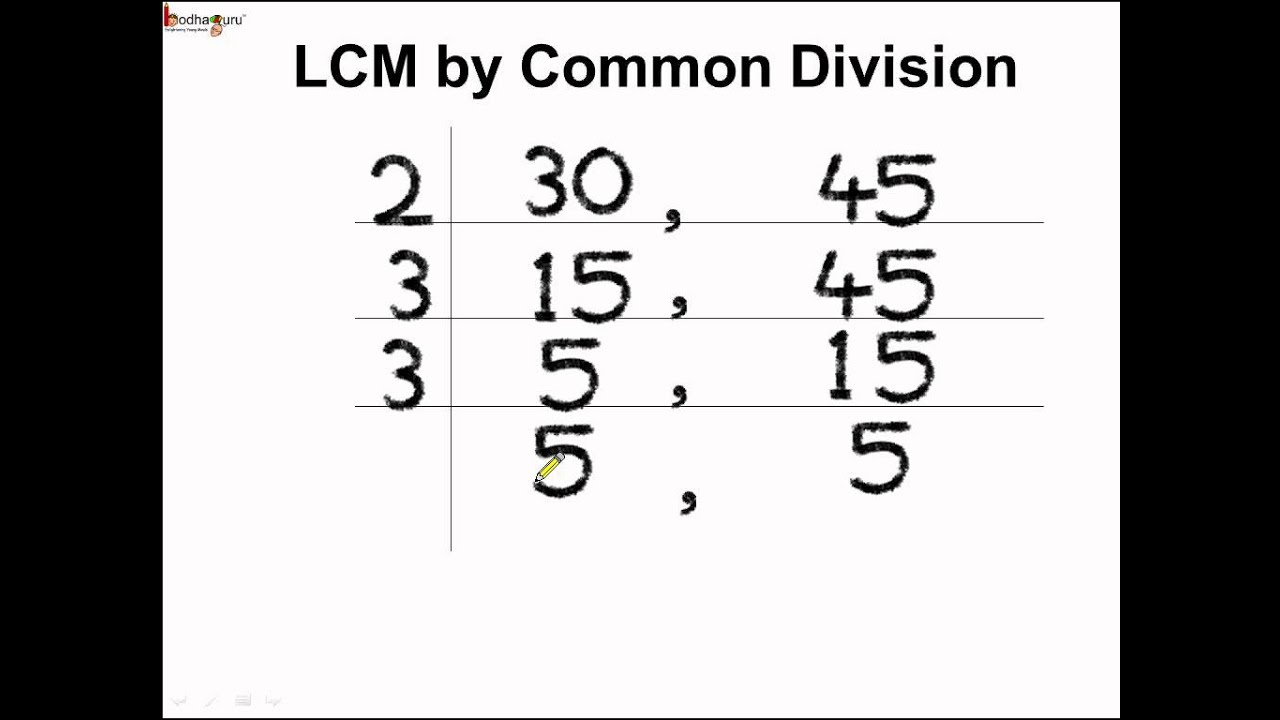
To find the LCM by division method, we write the given numbers in a row separately by commas, then divide the numbers by a common prime number. We stop dividing after reaching the prime numbers. The product of common and uncommon prime factor is the LCM of given numbers. Step 1: Write the given numbers in a horizontal line, separating them by commas. Step 2: Divide them by a suitable prime number, which exactly divides at least two of the given numbers. Step 3: We put the quotient directly under the numbers in the next row. If the number is not divided exactly, we bring it down in the next row. Step 4: We continue the process of step 2 and step 3 until all co-prime numbers are left in the last row. Step 5: We multiply all the prime numbers by which we have divided and the co-prime numbers left in the last row.

Lcm in division method

The least common multiple LCM of two numbers is the lowest possible number that can be divisible by both numbers. It can be calculated for two or more numbers as well. There are different methods to find the LCM of a given set of numbers. One of the quickest ways to find the LCM of two numbers is to use the prime factorization of each number and then the product of the highest powers of the common prime factors will be the LCM of those numbers.

Zillow barre vt

Solution: a. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. What is the LCM of 36 and 48? Maths Program. In this sense, a factor is also known as a divisor. Saudi Arabia. For this, we need to know the prime factors of the given numbers. The number itself. We copy down 31 to the next row and get 27 below How to find the LCM of numbers using the prime factorization method? How to find the HCF of numbers using the prime factorization method? Explanation: We only get the divisor and the quotients while doing the division method for finding the LCM. Explanation: To find the LCM using the long division method, divide 62 and by the prime number and obtain a quotient that can be further divided by the prime number.

Have you ever heard about LCM? The lowest number that can be divided by both numbers is known as the least common multiple LCM of two numbers.

True, the LCM of 12 and 15 is Multiply all divisors to get the HCF of given numbers. Given natural numbers to be written as the product of prime factors. In this method, divide the given numbers by common prime number until the remainder is a prime number or one. The LCM of 12 and 15 is Ans: True, in order to find the LCM by division method, we find a common prime number that divides the given numbers. Therefore, the LCM of 62 and by division method is Solution: a. In the second row, write the quotient we get after the division of 24 by 2. Solution: First let us find the LCM of 12 and 18 using the steps discussed below. Since only 6 is divisible by 2, we write the quotient and copy 9 as it is in the next row to get 3 and 9. The last divisor will be the HCF of given numbers.