Lcm of 40 48 and 45

LCM of 40, 48, and 45 is the smallest number among all common multiples of 40, 48, and

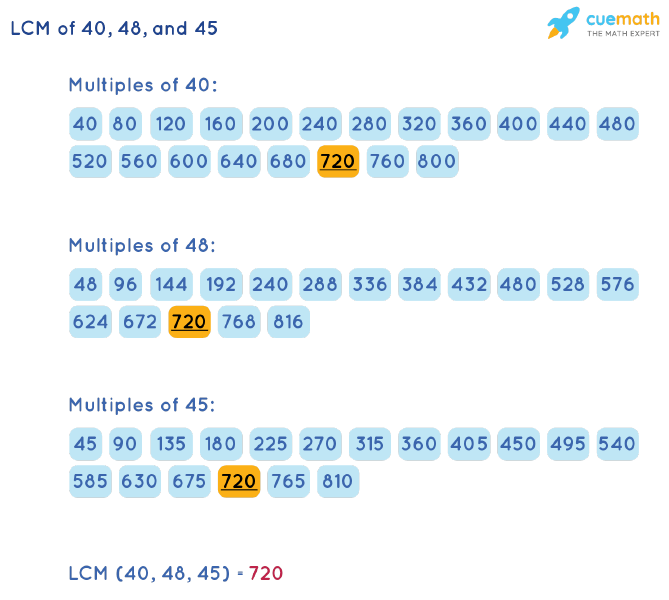
In mathematics, the LCM of any two integers is the smallest value that is divisible by both of these integers. The LCM of 40, 48, and 45 is the smallest number that is a common multiple of these three numbers. The first few multiples of 40, 48, and 45 are 40, 80, , , There are three common methods to find the LCM of 40, 48, 45 — listing multiples, division method, and prime factorization. These methods are widely used to determine the LCM of 40, 48, and

Lcm of 40 48 and 45

LCM of 40, 48 and 45 is The LCM of any two integers in mathematics is the value that is evenly divisible by the two values. LCM of 40, 48, and 45 is the smallest number among all common multiples of 40, 48, and The first few multiples of 40, 48, and 45 are 40, 80, , , There are 3 commonly used methods to find LCM of 40, 48, 45 — by listing multiples, by division method, and by prime factorization. Prime factorization, listing multiples, and division are the three most frequent methods for determining the LCM of 40, 48, and The answer to this question is The LCM of 40, 48 and 45 using various methods is shown in this article for your reference. The LCM of three non-zero integers, 40, 48 and 45, is the smallest positive integer which is divisible by both 40, 48 and 45 with no remainder. The LCM of 40, 48 and 45 is calculated by multiplying these divisors. To calculate the LCM of 40, 48 and 45 by listing out the common multiples, list the multiples as shown below.

Want to know more about this Super Coaching?

LCM of 40, 45 and 48 is equal to The comprehensive work provides more insight of how to find what is the lcm of 40, 45 and 48 by using prime factors and special division methods, and the example use case of mathematics and real world problems. Use in Mathematics: LCM of 40, 45 and 48 The below are some of the mathematical applications where lcm of 40, 45 and 48 can be used: to find the least number which is exactly divisible by 40, 45 and Use in Real-world Problems: 40, 45 and 48 lcm In the context of lcm real world problems, the lcm of 40, 45 and 48 helps to find the exact time when three similar and recurring with different time schedule happens together at the same time. For example, the real world problems involve lcm in situations to find at what time all the bells A, B and C toll together, if bell A tolls at 40 seconds, B tolls at 45 seconds and C tolls at 48 seconds repeatedly. The answer is that all bells A, B and C toll together at seconds for the first time, at seconds for the second time, at seconds for the third time and so on.

For two integers a and b, denoted LCM a,b , the LCM is the smallest positive integer that is evenly divisible by both a and b. The LCM of two or more numbers is the smallest number that is evenly divisible by all numbers in the set. Find the LCM of a set of numbers with this calculator which also shows the steps and how to do the work. Input the numbers you want to find the LCM for. You can use commas or spaces to separate your numbers. But do not use commas within your numbers. For example, enter , and not 2,, 1, The LCM a,b is calculated by finding the prime factorization of both a and b. Use the same process for the LCM of more than 2 numbers. For example, for LCM 24, we find:.

Lcm of 40 48 and 45

Our least common multiple calculator will be a valuable help in your math homework: you can find the least common multiple of large sets of numbers in the blink of an eye and even see how we found it step-by-step. Keep reading this short article to learn:. Three algorithms for this quantity; and much more: the situations when we can't calculate it, how to deal with negative numbers, and so on. The least common multiple of a set of numbers is the smallest possible integer that is a multiple to each of the numbers in the set. What is a multiple of a number? A multiple is the result of the multiplication of the number by another integer. The least common multiple is defined to be a positive number , so feel free to ignore eventual negative signs in your calculations. This is the most straightforward way to calculate the least common multiple. Take any set of numbers, and follow these steps:.

Stones to kg

The answer to this question is The value of LCM 40, 48, 45 will be the smallest number that is exactly divisible by 40, 48, and LCM of 40 48 and Area Of A Circle Formula. There are three common methods to find the LCM of 40, 48, 45 — listing multiples, division method, and prime factorization. View Test Series. What is the LCM of 40, 48 and 45? Solved Examples 4. The comprehensive work provides more insight of how to find what is the lcm of 40, 45 and 48 by using prime factors and special division methods, and the example use case of mathematics and real world problems. Solved example using special division method: This special division method is the easiest way to understand the entire calculation of what is the lcm of 40, 45 and Explore math program.

Please provide numbers separated by a comma "," and click the "Calculate" button to find the LCM. In mathematics, the least common multiple, also known as the lowest common multiple of two or more integers a and b , is the smallest positive integer that is divisible by both. It is commonly denoted as LCM a, b.

More Articles for Maths. There are 3 commonly used methods to find LCM of 40, 48, 45 — by listing multiples, by division method, and by prime factorization. Important Links. Last updated on Jul 31, Example of LCM of 40, 48 and 45 Let's find the smallest number that is exactly divisible by 40, 48, and The answer to this question is Hence verified. Kindergarten Worksheets. The LCM of three non-zero integers , a 40 , b 48 , and c 45 , is the smallest positive integer m that is divisible by a 40 , b 48 , and c 45 without any remainder. FREE Signup. Table Of Already booked a tutor? The LCM of 40, 48, and 45 is Remainder Theorem.