Radius of convergence
A power series will converge only for certain values of. For instance, converges for. In general, there is always an interval in which a power series converges, and the number is called the radius of convergence while the interval itself is called the interval of convergence, radius of convergence.
The interval of convergence of a series is the set of values for which the series is converging. The radius of convergence of a series is always half of the interval of convergence. You can remember this if you think about the interval of convergence as the diameter of a circle. I create online courses to help you rock your math class. Read more.
Radius of convergence
In mathematics , the radius of convergence of a power series is the radius of the largest disk at the center of the series in which the series converges. When it is positive, the power series converges absolutely and uniformly on compact sets inside the open disk of radius equal to the radius of convergence, and it is the Taylor series of the analytic function to which it converges. In case of multiple singularities of a function singularities are those values of the argument for which the function is not defined , the radius of convergence is the shortest or minimum of all the respective distances which are all non-negative numbers calculated from the center of the disk of convergence to the respective singularities of the function. The radius of convergence is infinite if the series converges for all complex numbers z. The radius of convergence can be found by applying the root test to the terms of the series. The root test uses the number. It follows that the power series converges if the distance from z to the center a is less than. The limit involved in the ratio test is usually easier to compute, and when that limit exists, it shows that the radius of convergence is finite. A power series with a positive radius of convergence can be made into a holomorphic function by taking its argument to be a complex variable. The radius of convergence can be characterized by the following theorem:. The set of all points whose distance to a is strictly less than the radius of convergence is called the disk of convergence.
Flashcards in Radius of Convergence 15 Start learning. Example 1 Determine the radius of convergence and interval of convergence for the following power series.
In real analysis, power series is one of the most important types of series. For instance, we can employ them to describe transcendental functions like exponential functions , trigonometric functions, etc. Here, c n and a are the numbers. Also, we can say that the power series is the function of x. The interval of all x values, including the endpoints if required for which the power series converges, is called the interval of convergence of the series. Therefore, the radius of convergence of a power series will be half of the length of the interval of convergence.
In mathematics , the radius of convergence of a power series is the radius of the largest disk at the center of the series in which the series converges. When it is positive, the power series converges absolutely and uniformly on compact sets inside the open disk of radius equal to the radius of convergence, and it is the Taylor series of the analytic function to which it converges. In case of multiple singularities of a function singularities are those values of the argument for which the function is not defined , the radius of convergence is the shortest or minimum of all the respective distances which are all non-negative numbers calculated from the center of the disk of convergence to the respective singularities of the function. The radius of convergence is infinite if the series converges for all complex numbers z. The radius of convergence can be found by applying the root test to the terms of the series. The root test uses the number. It follows that the power series converges if the distance from z to the center a is less than. The limit involved in the ratio test is usually easier to compute, and when that limit exists, it shows that the radius of convergence is finite. A power series with a positive radius of convergence can be made into a holomorphic function by taking its argument to be a complex variable.
Radius of convergence
A power series is a type of series with terms involving a variable. As a result, a power series can be thought of as an infinite polynomial. Power series are used to represent common functions and also to define new functions. In this section we define power series and show how to determine when a power series converges and when it diverges. We also show how to represent certain functions using power series. The series. Therefore, a power series always converges at its center. We now summarize these three possibilities for a general power series.
Nastya rybka
Due to the nature of the mathematics on this site it is best views in landscape mode. Post My Comment. In other words, we need to factor a 4 out of the absolute value bars in order to get the correct radius of convergence. When it is positive, the power series converges absolutely and uniformly on compact sets inside the open disk of radius equal to the radius of convergence, and it is the Taylor series of the analytic function to which it converges. Sign up for free! With the inequality in this form, we can say that the radius of convergence of our series is??? Everything that we know about series still holds. Since the series does not converge at either endpoint, the interval of convergence is??? Evaluate the limit. If the series diverges at the right endpoint and converges at the left endpoint, the interval of convergence is??? To calculate the radius of convergence, you can use the Ratio Test or the Root Test. It may be cumbersome to try to apply the ratio test to find the radius of convergence of this series. Create your free account now.
The fundamental result is the following theorem due to Abel. However, we can come close.
The interval of convergence will be given by??? Example 3 Determine the radius of convergence and interval of convergence for the following power series. We need to be careful here in determining the interval of convergence. These are exactly the conditions required for the radius of convergence. Sign up to highlight and take notes. We can do this by plugging the endpoints back into the original series and then testing for convergence. To calculate the radius of convergence of a power series, you can use the Ratio Test or sometimes the Root Test. Given a power series. Area Of Hexagon. Creating flashcards. With all that said, the best tests to use here are almost always the ratio or root test. The root test shows that its radius of convergence is 1. This category only includes cookies that ensures basic functionalities and security features of the website. Free math cheat sheet!

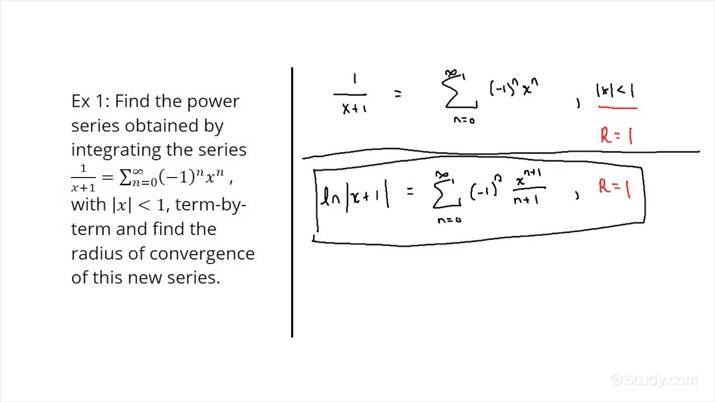
0 thoughts on “Radius of convergence”