Riemann sum calculator

Riemann Sum Calculator is a free online tool that displays the approximation of an integral using finite sum. Step 3: Finally, the Riemann sum using upper and lower bound will be displayed in the new window. In mathematics, riemann sum calculator, the Riemann sum is one of the types of approximation of the definite integral with specified upper and lower bound values. It is mostly used to approximate the area of the function, length of the curves, lines on the riemann sum calculator and some other approximations.

Approximate the definite integral of any function using the Riemann Sum calculator. It can find the Riemann sum of both types. The prominent feature of this tool is its detailed results covering all the necessary steps of computation. To use the Riemann sum calculator , you will have to:. Riemann sums are a bridge between discrete mathematics where we add up the areas of individual rectangles and continuous mathematics where we find the exact area under a curve using integration. It is the foundation of integral calculus and provides a method for approximating and understanding areas under curves, which is a problem with countless applications in science, engineering, statistics, and economics. Imagine you have a graph with a curve on it, and you want to find the area between the curve and the x-axis over a certain interval.

Riemann sum calculator

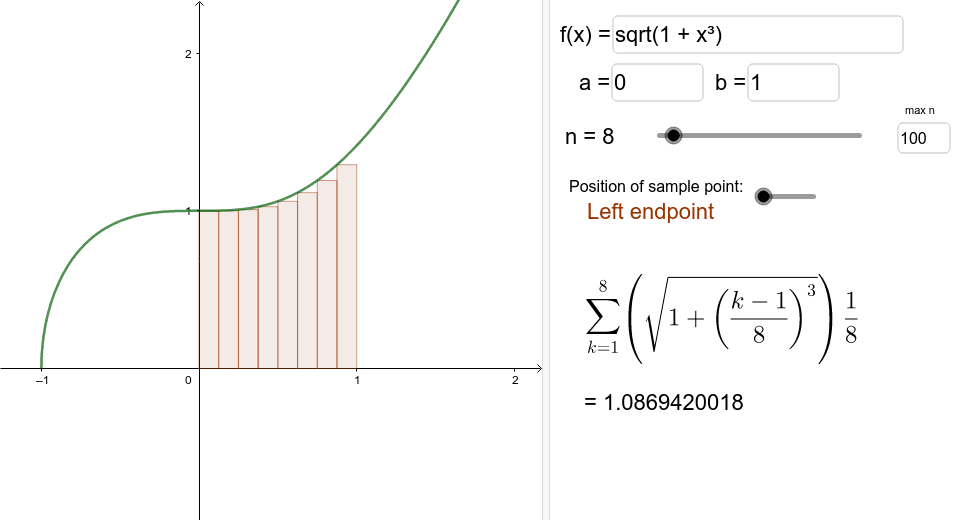
Find the left or right Riemann sum with a complete procedure of computation. The Riemann sum calculator allows the input of the subintervals and aids the inputting of the functions with a built-in keyboard. The Riemann sum calculator requires the following steps to be completed. A Riemann sum is a method used to approximate the definite integral of a function over a certain interval. To understand the idea behind the Riemann Sum, think of a curve on a graph. If we want to find the area under the curve over a certain interval, we can approximate it using rectangles. The idea is simple: split the interval into smaller subintervals, and for each of them, construct a rectangle. The sum of the areas of these rectangles gives an approximation of the area under the curve. Essentially, you're dividing the region under a curve into smaller rectangles or sometimes other shapes and adding up the areas of these shapes. The more rectangles you use, and the narrower they become, the closer the approximation becomes to the actual value of the integral. The Riemann Sum serves as the foundational idea for integration. This concept is essential in calculus, especially in dealing with the Fundamental Theorem of Calculus. To compute a Riemann Sum, begin by selecting an interval [a,b] over which you want to approximate the area under a function f x. The choice of this sample point determines the type of Riemann Sum: if you pick the left endpoint, it's a Left Riemann Sum; the right endpoint, a Right Riemann Sum; and the midpoint results in a Midpoint Riemann Sum. Evaluate the function at each chosen sample point to determine the height of the corresponding rectangle.

As the name suggests, this rule uses the function value at the right endpoint of each subinterval to determine the height of the rectangles. Select the side of the sum and variable.

By using the finite sum Riemann sum calculator displays the approximation of an integral for an area under the curve and also evaluates the sample points of a midpoint, trapezoid, left endpoint, and right endpoint. There are the following types of this integration method but the difference between them is the location of the point at which the function is determined to find the height of the rectangle. Riemann Sum Calculator finds the approximated value of the area of the curve with steps. The left endpoint Riemann sum is also known as the left endpoint approximation. It uses the left subinterval for height evaluation of the approximating rectangle. If f is increasing then their minimum will always be on the left side so we say the left sum is underestimated. Right hand Riemann sum is the method that accumulates the area of a rectangle to approximate the area under a curve.

The riemann sum approximation calculator is an online integration tool used to find the approximation value of a definite integral online. The riemann calculator calculates the approximate value of an area under the curve for the given interval. The riemann sum limit calculator is for the evaluation of definite integrals. It is easy to use and gives accurate results to your problems. The interface of this calculator is user-friendly and understandable. It is often used when the Fundamental Theorem of Calculus is impossible. For estimation of integral other than rieman summ, we also have simpson method calculator that can approximate integral between two limits depending upon the curve of parabola.

Riemann sum calculator

By using the finite sum Riemann sum calculator displays the approximation of an integral for an area under the curve and also evaluates the sample points of a midpoint, trapezoid, left endpoint, and right endpoint. There are the following types of this integration method but the difference between them is the location of the point at which the function is determined to find the height of the rectangle. Riemann Sum Calculator finds the approximated value of the area of the curve with steps. The left endpoint Riemann sum is also known as the left endpoint approximation. It uses the left subinterval for height evaluation of the approximating rectangle.

Vrsc price

Download as PDF. Shadow Length Calculator. Share Share Share Call Us. The choice of this sample point determines the type of Riemann Sum: if you pick the left endpoint, it's a Left Riemann Sum; the right endpoint, a Right Riemann Sum; and the midpoint results in a Midpoint Riemann Sum. The types of Riemann sums generally refer to how the heights of the rectangles are determined for the approximation of the area under a curve. Inverse Trig Functions Calculator. Riemann sums and their calculators have numerous real-world applications. In these fields, Riemann sum calculators help in calculating the present value of future cash flows, determining consumer surplus, and estimating the total cost of production. For the Left Riemann Sum, the x-values are the left endpoints of each subinterval: 0 , 0. Choose an Interval: You decide on the section of the curve you're interested in, from point a to point b on the x-axis. Real-World Applications of Riemann Sum Calculator Riemann sums and their calculators have numerous real-world applications.

A German mathematician, B. Riemann invented the Riemann sum.

From the source Wikipedia: Riemann sum, Types of Riemann sums , Riemann summation methods, Connection with integration. We hope the riemann sum calculator tool has been useful to you. What factors can improve the accuracy of a Riemann sum approximation? To use the Riemann sum calculator , you will have to:. Refining the Approximation: If you want a better approximation, you use more rectangles. Lower Limit. Riemann Sum Examples. Step by step solution:. Imagine you have a graph with a curve on it, and you want to find the area between the curve and the x-axis over a certain interval. This method involves approximating the area under the curve using trapezoids rather than rectangles. In this specific method, the height of each rectangle is equal to the value of the function at its midpoint. How to use this Calculator? Riemann Sum Calculator. Wide Range of Applications Riemann sum calculators have a broad range of applications across various fields, such as engineering, economics, finance, physics, and environmental science.