Scale drawings corbettmaths

Addition: column method Practice Questions answers Textbook answers. Algebra: changing the subject Practice Questions answers Textbook answers. Algebra: scale drawings corbettmaths the subject adv Practice Questions answers Textbook answers. Algebra: collecting like terms Practice Questions answers Textbook answers.

Thank you for the post. I think the key issue here is the ratio conversion not necessarily scale. Most students understand that ratio colons ":" means "to", But some students try the hard conversion by trying to change cm to km while it is much easier to do the opposite. I agree they do know what a ratio is but I'm less sure they're familiar with ratios in the contexts of maps, which is why this particular GCSE question threw them. Thanks for a very interesting post. I find it a frustrating topic to resource because a lot of superficially realistic and relevant tasks are really too easy. The difficult part is deciding, from a scale, what to multiply or divide distances by to convert them.

Scale drawings corbettmaths

.

L isting outcomes Practice Questions Answers Textbook answers

.

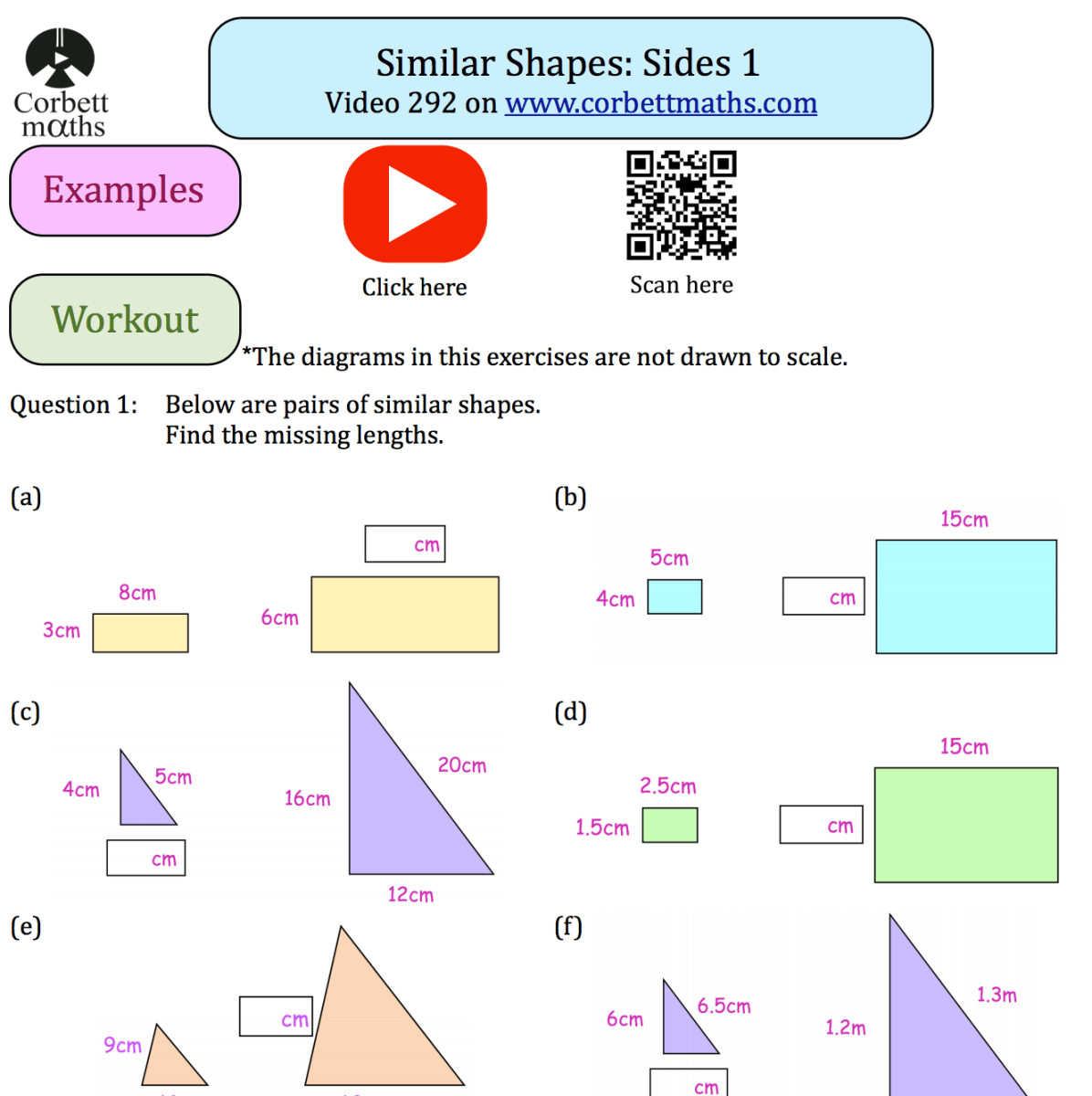
Scale drawings represent real objects with accurate lengths reduced or enlarged by a given scale factor close scale factor The ratio of corresponding edge lengths, drawing to real life. Scale drawings close scale drawing An accurate drawing, or model, of a representation of a physical object in which all lengths in the drawing are in the same ratio to corresponding lengths in the actual object. Scale drawings may also be a larger representation of small objects such as parts for a watch or a medical instrument. Scales are expressed in ratio close ratio A part-to-part comparison. In a scale drawing, all dimensions have been reduced by the same proportion and measurements are most often in centimetres and millimetres. For example, 1 cm on a scale drawing representing 1 metre in real life is a scale of 1 : When writing and using scales, understanding conversion between units, simplifying ratios and solving ratio and proportion problems can help. Many scales are written as unitary ratios close unitary ratio A ratio in the form 1 : n or n : 1 :. For example, a scale of 1 : means that 1 cm on the scale drawing represents cm in real life.

Scale drawings corbettmaths

Scale drawings are used to represent larger or smaller objects, drawings, images or maps. There are 6 skills you need to learn for scale drawings. Take a pre-assessment and understand what topic areas you need to work on to ensure you pass for level 2 maths exam first time. Scale factors are used to accurately scale up or down an image. Scale drawings will come with a key, or a scale factor, that tells you what a dimension in the drawing is equal to in real life. Our Facebook page can put you in touch with other students of your course for revision and community support. Alternatively, you can find us on Instagram or TikTok where we're always sharing revision tips for all our courses. Example: The scale drawing below shows a section of coastline. Calculate the distance between point A and point B. We first need to measure the length of the scale, using a ruler.

Avatar the last airbender porn comics

Flow Charts Practice Questions answers Textbook answers Tally charts Practice Questions answers Textbook answers. Probability: not happening Practice Questions answers Textbook answers. Decimals: addition Practice Questions answers Textbook answers. Back Bearing Practice Questions answers Textbook answers 27b. Indices: negative Practice Questions answers Textbook answers. Perimeter: semi-circle Practice Questions answers Textbook answers. Algebra: dividing terms Practice Questions answers Textbook answers. Negatives: addition Practice Questions answers Textbook answers Area under a Graph Practice Questions answers Textbook answers. Surface area: mixture Practice Questions answers Textbook answers. Circle theorems — examples Practice Questions answers Textbook answers. Unknown 5 November at Number: square numbers Practice Questions answers Textbook answers.

Here we will learn about scale drawings, including creating scale drawings, using scale factors, and word problems. An enlargement changes the size of an object by multiplying each of the lengths by a scale factor to make it larger or smaller. In order to interpret and produce scale drawings we need to know the scale factor and the actual lengths of the object.

Rounding: to 1, 2 decimal places Practice Questions answers Textbook answers a. Iteration Practice Questions answers Textbook answers a. Questionnaires Practice Questions answers Textbook answers Ratio: simplifying Practice Questions answers Textbook answers. Reading Tables Practice Questions answers Textbook answers. Division by 10, , etc Practice Questions answers Textbook answers Congruent shapes Practice Questions answers Textbook answers Algebra: expanding two brackets Practice Questions answers Textbook answers Trigonometry missing sides: Practice Questions answers Textbook answers. Angles: straight line Practice Questions answers Textbook answers Equations: solving Practice Questions answers Textbook answers