Sin3x differentiation
Derivative of sin3x sin3x differentiation 3cos3x. It is part of Differentiation which is a sub-topic of calculus. Sin3x is a composite function of two elementary functions namely, algebraic function and trigonometric function, sin3x differentiation. In the derivative of sin3x, 3x is a pure algebraic function whereas sin[f x ] is a trigonometric function.
The derivative of sin3x is equal to 3cos3x. We can evaluate the differentiation of sin3x using different methods of derivatives such as the first principle of derivatives and the chain rule method. We will also determine the formula for the derivative of sin3x using the first principle and the formula for the derivative of sin cube x and solve some examples related to the concept for a better understanding of the concept. Differentiation of sin3x is the process of finding its derivative which can be determined using various differentiation methods. We can find the derivative of sin3x using the first principle of derivatives, that is, the definition of limits and the chain rule method of differentiation. In the next section, let us explore the formula for the derivative of sin3x.
Sin3x differentiation
The chain rule is a tool for differentiating composite functions, that is, a function inside a function. Here, we have sin 3x. This can be thought of as the function 3x being put inside of the function sin x. When finding the derivative of such a function, the chain rule tells us that the derivative will be equal to the derivative of the outside function with the original inside function still inside of it, all multiplied by the derivative of the inside function. So, for sin 3x , the derivative the sin x , the outside function, is cos x. So, the first part of the chain rule, the differentiated outside function with the inside function unchanged, gives us cos 3x. Then, this is multiplied by the derivative of the inside function. What is the derivative of sin 3x? Jun 14, Explanation: The chain rule is a tool for differentiating composite functions, that is, a function inside a function. Related questions What is the Chain Rule for derivatives? See all questions in Chain Rule.
Terms and Conditions.
Note that in this post we will be looking at differentiating sin 3x which is not the same as differentiating sin 3 x. Here is our post dealing with how to differentiate sin 3 x. The chain rule is useful for finding the derivative of a function which could have been differentiated had it been in x, but it is in the form of another expression which could also be differentiated if it stood on its own. To perform the differentiation sin 3x , the chain rule says we must differentiate the expression as if it were just in terms of x as long as we then multiply that result by the derivative of what the expression is actually in terms of in this case the derivative of 3x. The Chain Rule: For two differentiable functions f x and g x.
The chain rule is a tool for differentiating composite functions, that is, a function inside a function. Here, we have sin 3x. This can be thought of as the function 3x being put inside of the function sin x. When finding the derivative of such a function, the chain rule tells us that the derivative will be equal to the derivative of the outside function with the original inside function still inside of it, all multiplied by the derivative of the inside function. So, for sin 3x , the derivative the sin x , the outside function, is cos x. So, the first part of the chain rule, the differentiated outside function with the inside function unchanged, gives us cos 3x. Then, this is multiplied by the derivative of the inside function.
Sin3x differentiation
The derivative of sin3x is equal to 3cos3x. We can evaluate the differentiation of sin3x using different methods of derivatives such as the first principle of derivatives and the chain rule method. We will also determine the formula for the derivative of sin3x using the first principle and the formula for the derivative of sin cube x and solve some examples related to the concept for a better understanding of the concept.
026009593
Maths Games. In this maths article we will learn how to differentiate sin3x by using various differentiation rules like the first principle of derivative and product rule. Our Team. To perform the differentiation sin 3x , the chain rule says we must differentiate the expression as if it were just in terms of x as long as we then multiply that result by the derivative of what the expression is actually in terms of in this case the derivative of 3x. The second derivative of sin3x is equal to -9 sin3x. The derivative of sin3x is equal to 3cos3x. Just be aware that not all of the forms below are mathematically correct. Example 2: Determine the second derivative of sin3x. Sri Lanka. We will use this fact as part of the chain rule to find the derivative of sin 3x with respect to x. Together it makes a composite function.
Learn what is the derivative of sin 3x with proof by first principle.
Report An Error. Then, this is multiplied by the derivative of the inside function. Sin3x is a composite function of two elementary functions namely, algebraic function and trigonometric function. Math is a life skill. Maths Program. United States. The derivative of sin3x is equal to 3cos3x. Together it makes a composite function. Our Journey. In this case: We know how to differentiate sin x the answer is cos x We know how to differentiate 3x the answer is 3 This means the chain rule will allow us to differentiate the expression sin 3x. Explore math program. Maths Games. The first derivative is obtained by applying the product rule. Maths Formulas.

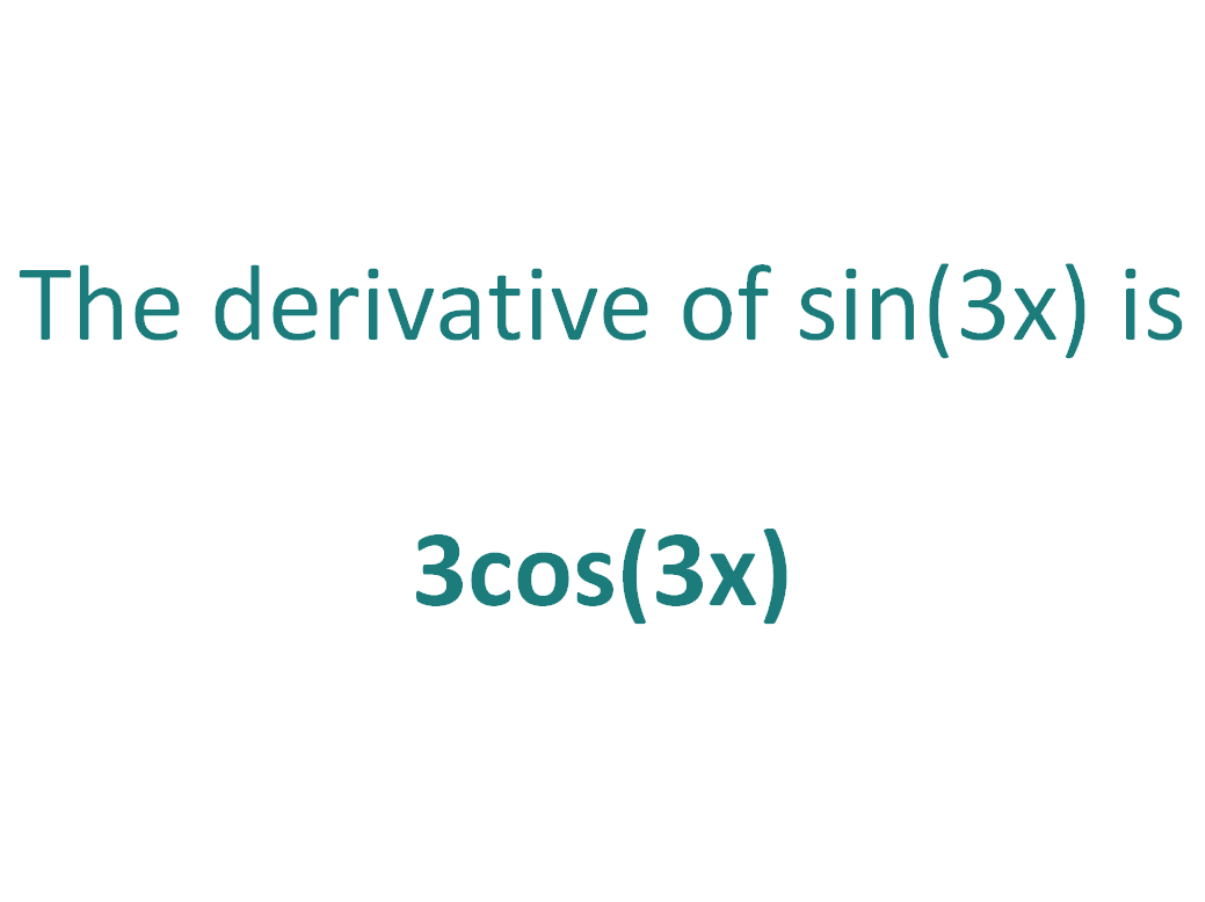
Bravo, magnificent idea