Slope of a line passing through two points

The equation of a line is an algebraic method to represent a set of points that together form a line in a coordinate system. The various points that together form a line in the coordinate axis can be represented as a set of variables x, y in order to form an algebraic equation, also referred to as the equation of a line.

The slope calculator determines the slope or gradient between two points in the Cartesian coordinate system. The slope is basically the amount of slant a line has and can have a positive, negative, zero, or undefined value. Before using the calculator, it is probably worth learning how to find the slope using the slope formula. To find the equation of a line for any given two points that this line passes through, use our slope intercept form calculator. Here, we will walk you through how to use this calculator, along with an example calculation, to make it simpler for you.

Slope of a line passing through two points

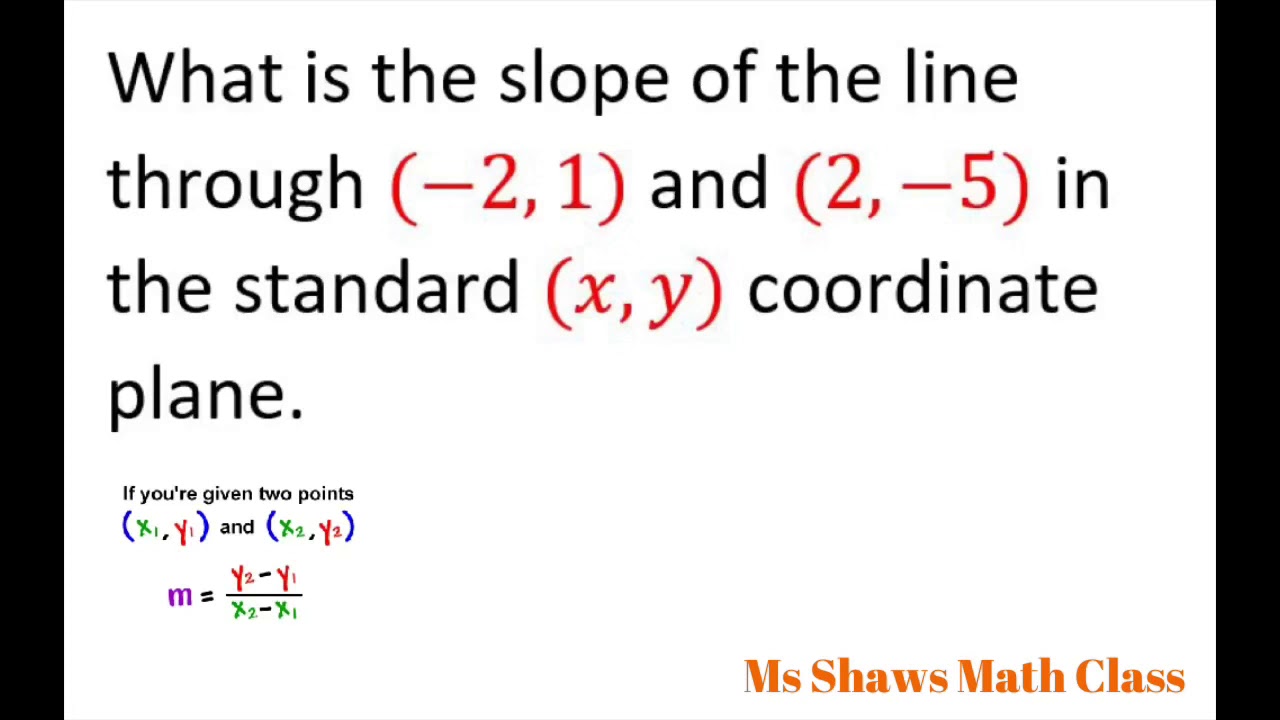
Sometimes we need to find the slope of a line between two points and we might not have a graph to count out the rise and the run. We could plot the points on grid paper, then count out the rise and the run, but there is a way to find the slope without graphing. Before we get to it, we need to introduce some new algebraic notation. But when we work with slopes, we use two points. Mathematicians use subscripts to distinguish between the points. A subscript is a small number written to the right of, and a little lower than, a variable. You can also find the slope of a straight line without its graph if you know the coordinates of any two points on that line. Consider two points on a line—Point 1 and Point 2. Since we have two points, we will use subscript notation. So we can rewrite the rise using subscript notation. So we rewrite the run using subscript notation. We can use this formula to find the slope of a line when we have two points on the line.

A line segment can be defined as a connection between two points. Sphere Volume Calculator.

The slope of a line is its vertical change divided by its horizontal change, also known as rise over run. When you have 2 points on a line on a graph the slope is the change in y divided by the change in x. Input two points using numbers, fractions, mixed numbers or decimals. The slope calculator shows the work and gives these slope solutions:. You will also be provided with a custom link to the Midpoint Calculator that will solve and show the work to find the midpoint and distance for your given two points. Here you need to know the coordinates of 2 points on a line, x 1 , y 1 and x 2 , y 2. Say you know two points on a line and their coordinates are 2, 5 and 9,

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Learn how to write the slope formula from scratch and how to apply it to find the slope of a line from two points. It's kind of annoying to have to draw a graph every time we want to find the slope of a line, isn't it? We can avoid this by writing a general formula for slope. Before we start, let's remember how slope is defined:.

Slope of a line passing through two points

The point-slope form calculator will show you how to find the equation of a line from a point on that line and the line's slope. Soon, you will know what is point-slope form equation, and learn how is it different from the slope-intercept form equation. We also came up with two exercises, and we'll explain how to solve them in the last paragraph. The slope, also known as the gradient, is the marker of a line's steepness. If it's positive, it means the line rises. If it's negative — the line decreases. If it's equal to zero, the line is horizontal. You can find the slope between two points by estimating rise over run — the difference in height over a distance between two points. To find the gradient of non-linear functions, you can use the average rate of change calculator. There is more than one way to form an equation of a straight line.

Licensed practical nurse jobs in alberta

The distance between the two points. Enter the x and y coordinates of the first point, followed by the x and y coordinates of the second one. FAQ How to find slope from an equation? Convert both measurements into the same units. There are two absolutely necessary requirements for forming the equation of a line, which are the slope of the line and any point on the line. The angle the line makes with respect to the x-axis measure anti-clockwise. Each tool is peer-reviewed by a trained expert and then proofread by a native speaker. Maths Questions. How do you calculate the length of a slope? Saudi Arabia. Rate of change is particularly useful if you want to predict the future of previous value of something , as, by changing the x variable, the corresponding y value will be present and vice versa.

The slope calculator determines the slope or gradient between two points in the Cartesian coordinate system. The slope is basically the amount of slant a line has and can have a positive, negative, zero, or undefined value.

Draw a horizontal and a vertical line from the two points A and B respectively such that they meet at C. Use either the point slope form or slope intercept form equation and work out the math to rearrange the equation into standard form. The distance between the two points. You can also use the distance calculator to compute which side of a triangle is the longest, which helps determine which sides must form a right angle if the triangle is right. If you know the slope of a line, any line parallel to it will have the same slope and these lines will never intersect. CC licensed content, Original. Rate of change can be found by dividing the change in the y vertical direction by the change in the x horizontal direction, if both numbers are in the same units, of course. Other related topics Just as slope can be calculated using the endpoints of a segment, the midpoint can also be calculated. Image will be Uploaded Soon. Add the two values together. Use the Pythagorean theorem to find the length of the slope. There are two absolutely necessary requirements for forming the equation of a line, which are the slope of the line and any point on the line. The larger the value is, the steeper the line. Breakdown tough concepts through simple visuals. This is true for all vertical lines—they all have a slope that is undefined.