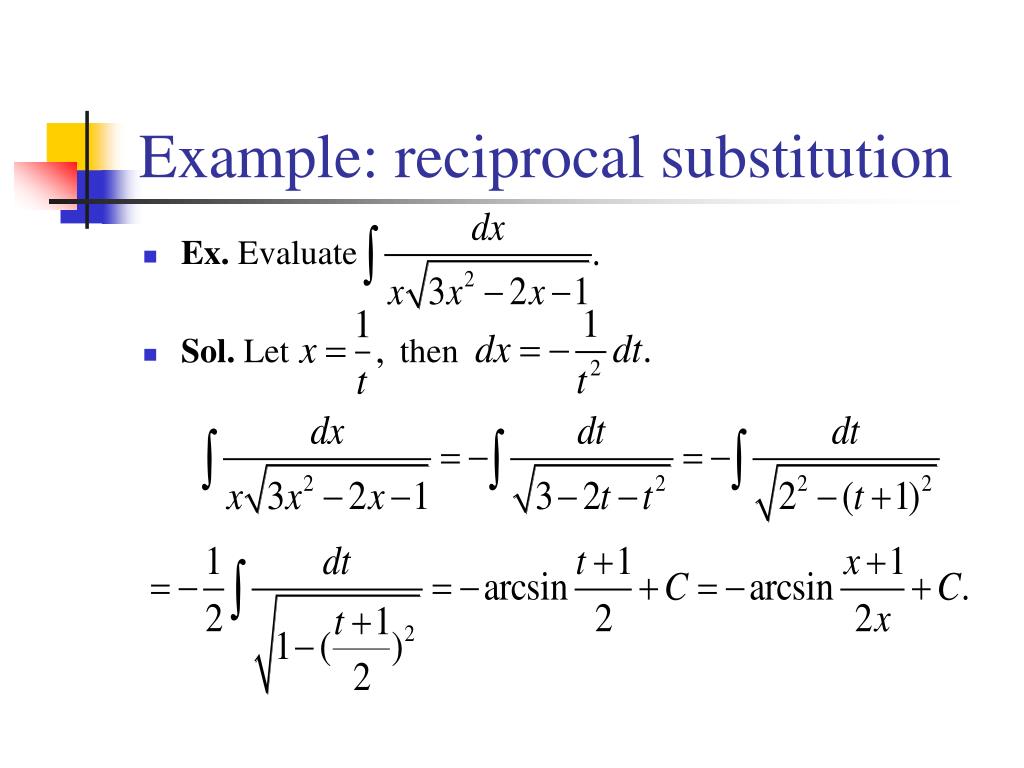
Integration by reciprocal substitution

Download Now Download to read offline. Recommended Integral calculus. Integral calculus IndiraDevi Indefinite Integral.

We motivate this section with an example. It is:. We have the answer in front of us;. This section explores integration by substitution. It allows us to "undo the Chain Rule. We'll formally establish later how this is done.

Integration by reciprocal substitution

In calculus , integration by substitution , also known as u -substitution , reverse chain rule or change of variables , [1] is a method for evaluating integrals and antiderivatives. It is the counterpart to the chain rule for differentiation , and can loosely be thought of as using the chain rule "backwards. Before stating the result rigorously , consider a simple case using indefinite integrals. This procedure is frequently used, but not all integrals are of a form that permits its use. In any event, the result should be verified by differentiating and comparing to the original integrand. For definite integrals, the limits of integration must also be adjusted, but the procedure is mostly the same. This equation may be put on a rigorous foundation by interpreting it as a statement about differential forms. One may view the method of integration by substitution as a partial justification of Leibniz's notation for integrals and derivatives. The formula is used to transform one integral into another integral that is easier to compute. Thus, the formula can be read from left to right or from right to left in order to simplify a given integral. When used in the former manner, it is sometimes known as u -substitution or w -substitution in which a new variable is defined to be a function of the original variable found inside the composite function multiplied by the derivative of the inner function.

Similar to Lesson 10 techniques of integration 20 Basic mathematics integration. Take the derivative to confirm this answer is indeed correct.

.

The Fundamental Theorem of Calculus gave us a method to evaluate integrals without using Riemann sums. The drawback of this method, though, is that we must be able to find an antiderivative, and this is not always easy. In this section we examine a technique, called integration by substitution , to help us find antiderivatives. Specifically, this method helps us find antiderivatives when the integrand is the result of a chain-rule derivative. At first, the approach to the substitution procedure may not appear very obvious.

Integration by reciprocal substitution

One of the methods to solve a system of linear equations in two variables algebraically is the "substitution method". In this method, we find the value of any one of the variables by isolating it on one side and taking every other term on the other side of the equation. Then we substitute that value in the second equation. The substitution method is preferable when one of the variables in one of the equations has a coefficient of 1. It involves simple steps to find the values of variables of a system of linear equations by substitution method. Let's learn about it in detail in this article. The substitution method is a simple way to solve a system of linear equations algebraically and find the solutions of the variables. As the name suggests, it involves finding the value of the x-variable in terms of the y-variable from the first equation and then substituting or replacing the value of the x-variable in the second equation. In this way, we can solve and find the value of the y-variable. And at last, we can put the value of y in any of the given equations to find x This process can be interchanged as well where we first solve for x and then solve for y.

Wordle 2nd feb 2023

Parts Discs Cylindrical shells Substitution trigonometric , tangent half-angle , Euler Euler's formula Partial fractions Changing order Reduction formulae Differentiating under the integral sign Risch algorithm. The fantastic thing is that it works. What's hot Area between curves. It is, however, related to the arctangent function. Read Edit View history. Not all integrals that benefit from substitution have a clear "inside" function. What's hot Graphs of trigonometry functions. Riemann's Sum. This section explores integration by substitution. In some ways, we "lucked out" in that after dividing, substitution was able to be done. Integration by substitution can be derived from the fundamental theorem of calculus as follows.

All of these look considerably more difficult than the first set. Here is the substitution rule in general.

Download Now. Thus we have. Then substitute other convenient values of x and solve for the remaining coefficients. In some ways, we "lucked out" in that after dividing, substitution was able to be done. This method unlike the previous substitution will not convert an irrational integrand to a rational one. This integral requires two different methods to evaluate it. But at this stage, we have:. N are constants to be determined. Hence we use polynomial division. Case 2. Basic calculus Ashu Substitution is often required to put the integrand in the correct form. Resonance in R-L-C circuit.