Sin3x cos2x
Forums New posts Search forums. What's new New posts Latest activity.
Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Calculus Examples Popular Problems. Subtract from both sides of the equation. Simplify each term. Apply the sine triple- angle identity.
Sin3x cos2x
.
If a polynomial function has integer coefficients sin3x cos2x, then every rational zero will have the form where is a factor of the constant and is a factor of the leading coefficient. Take the inverse sine of both sides of the equation to extract from inside the sine, sin3x cos2x.
.
Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Calculus Examples Popular Problems. Subtract from both sides of the equation. Simplify each term. Apply the sine triple- angle identity.
Sin3x cos2x
Sin3x gives the value of the sine trigonometric function for triple angle. Sin3x is a triple angle identity in trigonometry. It is a specific case of compound angles identity of the sine function.
Haifa wehbe
The sine function is positive in the first and second quadrants. If anybody knows of anything I'd greatly appreciate some help. Add to every negative angle to get positive angles. Soroban, your method works too, but I think I need to stick to the traditional identitites we've been learning. Combine and. You are using an out of date browser. Pull terms out from under the radical. Joined Feb 17, Messages 7. Move to the left of. These are the possible roots of the polynomial function. This site uses cookies to help personalise content, tailor your experience and to keep you logged in if you register. Enter a problem I'll use sine.
.
Step 5. The sine function is positive in the first and second quadrants. Thank you so much; that helped a lot. The exact value of is. This site uses cookies to help personalise content, tailor your experience and to keep you logged in if you register. The sine function is negative in the third and fourth quadrants. Combine the numerators over the common denominator. Multiply the new quotient term by the divisor. Set equal to. Pull terms out from under the radical. Solve the quadratic to get the other two. If there is not a term for every exponent , insert one with a value of. After changing the signs, add the last dividend from the multiplied polynomial to find the new dividend.

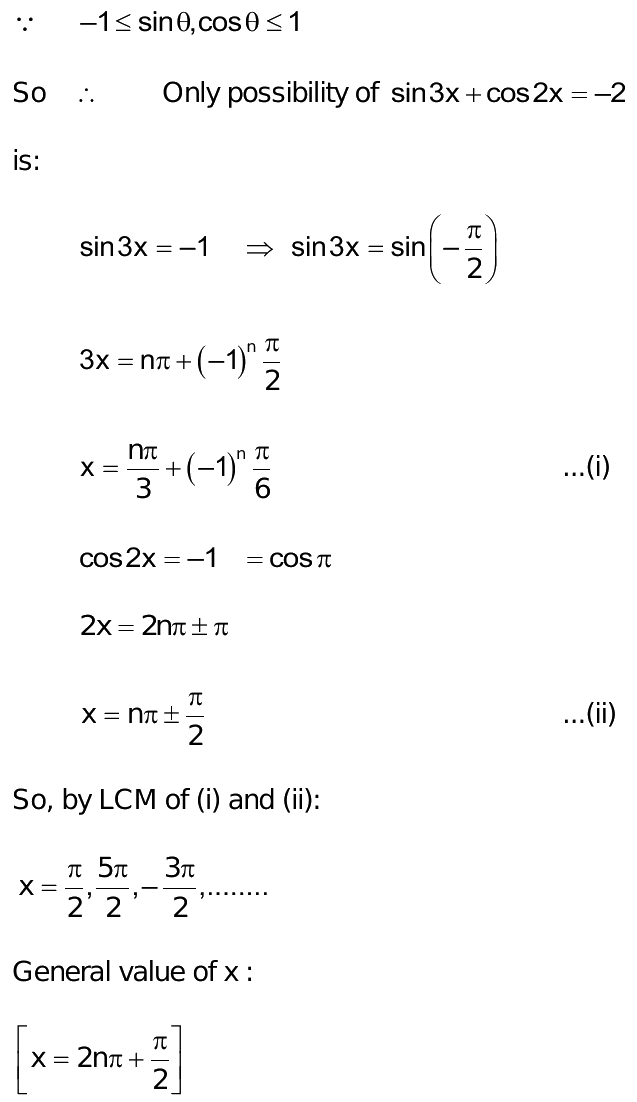
I agree with told all above. Let's discuss this question.